A flowchart proof brainlycraigslist buena vista investigation reveals a fascinating intersection of online learning resources and geographical context. This study explores how students in Buena Vista might leverage platforms like Brainly, a question-and-answer site, and Craigslist, a classifieds website, to access assistance with constructing geometric flowchart proofs. We examine the potential search patterns, the type of information available, and the impact of location on resource accessibility.
The research delves into the practical application of flowchart proofs in geometry, comparing them to traditional two-column proofs. It further analyzes how the unique characteristics of Buena Vista, potentially a smaller community, influence access to tutors and online learning materials compared to larger metropolitan areas. Hypothetical scenarios illustrate a student’s journey in seeking help, highlighting the strengths and limitations of each resource.
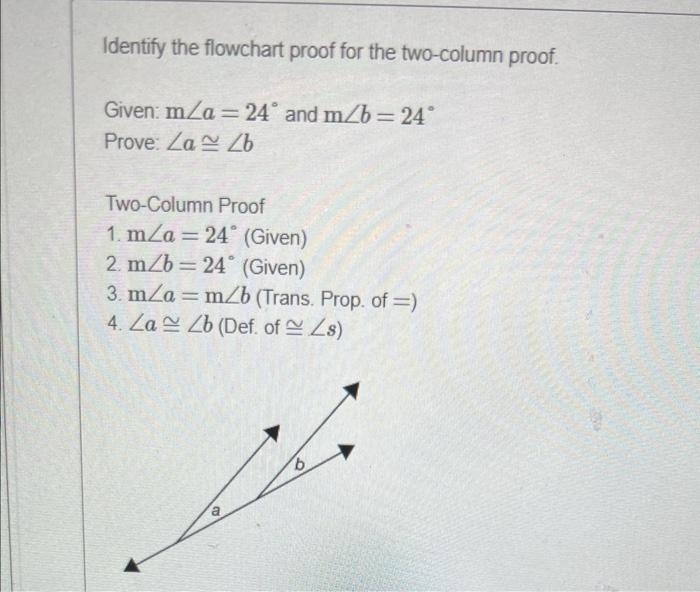
Flowchart Proofs in Geometry
Flowchart proofs offer a visual and organized approach to demonstrating geometric theorems, making complex logical arguments easier to understand and follow. This method utilizes a step-by-step process represented graphically, contrasting with the traditional two-column proof format. The use of flowcharts enhances comprehension, particularly for visual learners.
Fundamental Principles of Flowchart Proofs
Flowchart proofs in geometry rely on the sequential application of postulates, theorems, and definitions to arrive at a logical conclusion. Each step in the flowchart represents a logical deduction based on previously established facts or given information. The flow progresses linearly, with each step building upon the previous one until the desired conclusion is reached. Symbols and concise statements are used to represent geometric relationships and logical inferences.
Examples of Geometric Proofs Represented Using Flowcharts
Various geometric proofs can be effectively illustrated using flowcharts. These include proofs involving congruent triangles (SSS, SAS, ASA, AAS), similar triangles, parallel lines and transversals, and properties of circles. For example, a flowchart can elegantly demonstrate that the base angles of an isosceles triangle are congruent. Another example could be proving the theorem that the diagonals of a parallelogram bisect each other.
Constructing a Flowchart Proof
Constructing a flowchart proof involves a systematic approach. First, clearly state the given information and the theorem to be proven. Next, identify the necessary postulates, theorems, or definitions that will be used in the proof. Then, arrange these steps logically in a flowchart, using appropriate symbols to represent logical connections (e.g., arrows for implication, diamonds for conditional statements).
Each step should be clearly labeled and justified with a brief explanation. Finally, verify the logical flow of the proof to ensure that each step leads logically to the next.
Flowchart Proof of the Pythagorean Theorem
A flowchart proof for the Pythagorean Theorem (a² + b² = c²) would begin with the given right-angled triangle with sides a, b, and hypotenuse c. Subsequent steps would involve constructing squares on each side, employing area relationships, and ultimately demonstrating the equality a² + b² = c². The flowchart would visually trace the logical steps, showing how the area relationships lead to the theorem’s conclusion.
Comparison of Flowchart and Two-Column Proofs
Source: cheggcdn.com
Flowchart proofs offer a visual advantage over traditional two-column proofs, making the logical flow more apparent. However, two-column proofs might be more concise for simpler theorems. Flowchart proofs excel in demonstrating complex logical chains, while two-column proofs might be preferred for straightforward arguments. The choice depends on the complexity of the theorem and the learner’s preference.
Brainly and Craigslist: Educational Resource Search
Brainly and Craigslist, while seemingly disparate platforms, can indirectly intersect in a student’s search for help with geometry assignments. Brainly offers a question-and-answer forum, while Craigslist provides a platform for classified ads, including tutoring services.
Brainly’s Relevance to Flowchart Proofs
Brainly provides a platform where students can ask questions about flowchart proofs, access solutions to similar problems, and engage in discussions with peers and tutors. Searches on Brainly for “flowchart proof,” “geometry flowchart proof,” or specific geometric theorems combined with “flowchart proof” would yield relevant results. Students can find explanations, examples, and step-by-step solutions to various geometric problems using this method.
Craigslist’s Indirect Relation to Geometry Proof Resources
Craigslist can be used to find local tutors specializing in geometry who may be able to assist with flowchart proofs. Students might search for terms like “geometry tutor,” “math tutor,” or “high school math tutor,” specifying their need for help with proofs. The ads may include details about tutoring methods, including whether the tutor uses flowchart proofs in their instruction.
Hypothetical Scenario: Student Using Brainly and Craigslist
Imagine a student struggling with a flowchart proof involving similar triangles. They might first search Brainly for “flowchart proof similar triangles,” finding example problems and explanations. If still unsure, they could then turn to Craigslist, searching for “geometry tutor” in their local area (Buena Vista, in this case), contacting tutors who mention experience with flowchart proofs.
Information Found on Brainly Regarding Flowchart Proofs
On Brainly, a student could find a variety of resources, including: solved examples of flowchart proofs for various geometric theorems, explanations of the principles behind flowchart proofs, discussions among students and tutors regarding the use of flowcharts in geometry, and links to external resources providing further information on the topic.
Buena Vista and Geographic Context: Access to Educational Resources: A Flowchart Proof Brainlycraigslist Buena Vista
The geographic location of Buena Vista significantly impacts a student’s access to educational resources, including tutoring and online learning materials. Comparing Buena Vista to a larger metropolitan area highlights the disparities in resource availability.
Influence of Location on Resource Availability, A flowchart proof brainlycraigslist buena vista
In a smaller town like Buena Vista, the availability of specialized tutors might be limited compared to a larger city. Access to in-person tutoring for flowchart proofs might depend on the presence of qualified math tutors in the area. Online resources, however, might offer a more equitable opportunity, regardless of location.
Comparison of Resource Accessibility
A larger metropolitan area generally offers a wider range of tutoring options, including specialized geometry tutors experienced with flowchart proofs. Online resources are equally accessible in both locations, but the availability of local support networks and in-person tutoring varies considerably.
Geographic Context’s Impact on Finding Assistance
A student in Buena Vista might face challenges finding local tutors familiar with flowchart proofs, requiring them to rely more heavily on online resources. This might involve greater self-reliance and a need for more independent learning strategies.
Discussions on a flowchart proof found on Brainly Craigslist Buena Vista often involve logistical planning, mirroring the complexities of self-sufficiency. For those considering such a lifestyle, research into the best location is crucial; consider consulting resources like this Reddit thread on the best state for off grid living reddit to inform your decision-making process before embarking on such a project.
Ultimately, understanding the variables, much like constructing a sound flowchart proof, is key to success.
Hypothetical Study Comparing Online Resource Usage
A hypothetical study comparing online resource usage in Buena Vista and a larger city like Denver, Colorado, could reveal differences in the types of resources accessed (e.g., reliance on video tutorials versus interactive online exercises), frequency of online interaction, and overall success rates in understanding flowchart proofs.
Comparison of Resource Availability: Buena Vista vs. Denver
| Location | Availability of Tutors | Online Resources | Accessibility of Educational Materials |
|---|---|---|---|
| Buena Vista | Limited; Primarily general math tutors | Equally accessible as Denver | Moderate; limited local support |
| Denver | Abundant; Specialized geometry tutors readily available | Equally accessible as Buena Vista | High; extensive local support and resources |
Visual Representation and Illustration of Flowchart Proofs
Visual aids significantly enhance understanding of flowchart proofs. Detailed descriptions and illustrative examples are crucial for effective learning.
Detailed Description of a Flowchart Proof
Consider proving that vertically opposite angles are equal. The flowchart would begin with two intersecting lines, labeling the angles formed. The next step would involve stating the linear pair postulate, showing that adjacent angles add up to 180 degrees. Subsequent steps would use the transitive property to demonstrate the equality of vertically opposite angles. Each step would be clearly labeled and justified.
Flowchart for Solving a Geometric Problem
To solve a problem involving finding the missing angle in a triangle given two angles, the flowchart would begin by stating the given angles. The next step would utilize the triangle angle sum theorem (angles add up to 180 degrees). The flowchart would then demonstrate the calculation of the missing angle, culminating in the final answer.
Visual Aid for Enhancing Understanding
A diagram showing the intersecting lines and the labeled angles would enhance the flowchart proof for vertically opposite angles. Color-coding the angles and using arrows to indicate relationships would further improve clarity. Similarly, for the triangle problem, a diagram of the triangle with the given angles would be beneficial.
Improving Clarity with Visual Representations
Visual representations such as diagrams, flowcharts, and color-coding improve clarity by providing a concrete visual representation of abstract geometric concepts. They aid in understanding the logical flow of the proof and the relationships between different geometric elements.
Illustration of Constructing a Perpendicular Bisector
An illustration would depict a line segment. The flowchart would begin with the construction of two arcs from each endpoint of the segment, intersecting above and below the line. Then, it would show drawing a line connecting the intersection points, creating the perpendicular bisector. The diagram would clearly show the arcs, intersection points, and the resulting perpendicular bisector.
Each step in the construction would be clearly labeled and linked to the corresponding step in the flowchart.
Closing Notes
In conclusion, the search for “flowchart proof” across platforms like Brainly and Craigslist, viewed within the specific context of a location like Buena Vista, underscores the evolving landscape of educational resource access. While online platforms offer vast potential, geographic limitations and varying levels of resource availability significantly influence a student’s ability to effectively learn and apply concepts like flowchart proofs.
Further research into the digital divide and equitable access to online educational resources is warranted.
